Conjugate Gears
GlossaryBase pitch—The pitch that is measured on the base circle. In the basic rack similar sides of the teeth consist of a series of equally spaced parallel lines, and the base pitch is the perpendicular distance between the lines. Basic rack—The rack or segment of a gear wheel with an infinite radius belonging to a system of gearing in which the tooth forms are conjugate. Its pitch line is straight. Bevel gear—A gear for connecting shafts whose axes intersect, and whose pitch surfaces are cones. The apexes of the cones coincide at the intersection of the axes of the shafts, and their surfaces touch along a line lying in the plane containing the axes. Common connecting line—A straight line connecting the axes of bevel, hypoid, and worm gears and passing through the pitch point of conjugate gears. Common line of centers—A straight line joining the axes of a spur, helical, and crossed-axis helical gears, which is perpendicular to both axes, and is the shortest distance between the axes. Common normal—A straight line passing through a point of contact in a direction perpendicular to the tangent plane between the surfaces. Common tangent plane—The plane passing through a point of contact on two curved surfaces and is tangent to both surfaces. Conjugate action—The property of conjugate gears that produces a constant angular velocity ratio during meshing. Conjugate—Gear tooth profiles that produce a constant angular velocity ratio during meshing. Contact line—The effective line or curve of contact between mating gear teeth without rotation of the gears. Spur gears have contact lines that are parallel to the gear axis. Helical gears have straight contact lines that are inclined to the gear axis. Straight bevel and skew bevel gears have straight contact lines that consist of a cone element. Zerol, spiral bevel, hypoid, and worm gears have curved contact lines. Contact plane—See surface of action. Contact point—The point at which meshing gear teeth touch each other. Crossed-axis helical gear—Gears that connect shafts that are inclined to one another, but whose axes do not intersect. They are distinguished from hypoid gears in that their pitch surfaces are cylinders. Crossing points—The ends of a common connecting line that joins the axes of a pair of gears. Generatrix—A point, curve, or surface that, when moved along a given path, generates a new shape. Helical gear—A gear that has teeth in the form of a helix or screw. Helical gears connect parallel shafts. Helicoid—The tooth surface of screws, worms, and helical gears. Hypoid gear—A gear similar to a bevel gear, but one that operates on offset axes. Involute helicoid—A surface that is geometrically the same as the tooth surface of a helical gear. Its generatrix is tangent to a base cylinder that is concentric to the gear axis. Its tooth profile is an involute curve in the plane of rotation and an axvolute in the axial plane. Line of action—A straight line, normal to both mating tooth profiles at the point of contact that passes through the pitch point. Line of centers—See Common Line of Centers. Normal base pitch—The base pitch in the normal plane. It is the perpendicular distance between the parallel planes forming the sides of adjacent involute gear teeth of the basic rack. To be conjugate, any two gears must have the same normal base pitch. Path of contact—The locus of all points of contact between meshing gear tooth profiles. Pitch—The spacing between a point on one tooth and the corresponding point on an adjacent tooth. Pitch circle—A theoretical circle, concentric with the gear axis, having a diameter equal to the pitch cylinder. Pitch cone—The pitch surface of a bevel gear. Pitch cylinder—The pitch surface of a spur, helical, or crossed-axis helical gear. Pitch element—The line of tangency between two contacting pitch surfaces. It is the instantaneous axis of rotation. See common connecting line. Pitch line—A line tangent to the pitch circle at the pitch point. It is the line in the basic rack that rolls with the gear pitch circle without slipping. Pitch plane—The imaginary surface in a rack or generating crown gear that rolls without slipping with a pitch cylinder or pitch cone of a mating gear. It is tangent to the pitch surfaces and contains the pitch element. Pitch point—A fixed point in the common connecting line at which the pitch surfaces touch each other. In a hypoid gear, this is only meaningful at the mean point. Pitch surface—For parallel-axis spur and helical gears, and intersected-axis bevel gears, the imaginary planes, cylinders, or cones that roll together without slipping. Pressure angle—The angle between the line of action and the pitch line. It is so named because it indicates the direction of the pressure or force between meshing teeth. Profile shift—The amount that the pitch line of the basic rack cutter is shifted away from the gear reference circle. Reference circle (or cylinder)—The pitch circle or cylinder of engagement with the basic rack. Screw helicoid—A surface generated by a straight-line generatrix that intersects the axis of the helicoid. It is the form of a common screw thread. Also known as an Archimedean screw. Shaft angle—The angle between the axes of two non-parallel gear shafts. Spur gear—A gear having a cylindrical pitch surface and teeth that are parallel to its axis. Surface of action—The imaginary surface composed of all positions of the lines of contact. For parallel-axis spur and helical gears, and intersected-axis bevel gears, the surface of action is a plane surface that is tangent to either the base cylinders or the base cones. Crossed-axis helical gears have point contact and a line of action. Worm gears and hypoid gears have a warped surface of action. Tangent plane—The plane tangent to the tooth surfaces at a point or line of contact. Transmission error—The deviation of the position of a driven gear, for a given angular position of a driving gear, from the position that the driven gear would occupy if the gears were conjugate. Worm—A gear in the form of a screw thread, having one or more starts. Worm wheel—A special gear for engaging with a worm. |
Introduction
The conjugacy of meshing gears is one of the most important attributes of gears because it ensures a constant velocity ratio that gives smooth, uniform transmission of motion and torque. Some of the world’s greatest gear theoreticians like Earle Buckingham (Ref. 1), Wells Coleman (Ref. 2), and John Colbourne (Ref. 3) laid the foundation for understanding conjugacy. Their teachings and interpretations of the law of gearing have been used by generations of gear engineers to design and manufacture gear transmissions for almost everything that is mechanically actuated. The law of conjugate gear-tooth action according to Earle Buckingham (Ref. 1) is:
“To transmit uniform rotary motion from one shaft to another by means of gear teeth, the normals to the profiles of these teeth at all points of contact must pass through a fixed point in the common center line of the two shafts.”
In this paper, the authors use graphics and written explanations to present the requirements for conjugacy for the following involute-based gear types: spur, helical, crossed-axis helical, worm, bevel, and hypoid. Here the authors generalize the law of gearing such that it applies to all forms of conjugate gears:
Conjugate gears transmit uniform rotary motion from one shaft to another by means of gear teeth. The common normal to the profiles of these teeth, at all points of contact, must pass through a fixed point P in the common connecting line that intersects the two shaft axes and is normal to the pitch element.
During the past 50 years, sophisticated computer software for the design of gears has been developed. However, today, some gear engineers might take the results of these software systems for granted without recognizing the details upon which conjugacy in some cases depends.
With a knowledge of the requirements for conjugacy, the gear engineer can choose the right type of gear for a particular application, for example, spur versus helical, crossed-axis helical versus worm, and worm versus high-ratio hypoid gears.
However, if the gears are conjugate only under ideal operating conditions, they are not likely to remain conjugate when they are subjected to actual operating conditions of load, speed, temperature, and manufactured accuracy. Therefore, it is necessary to modify the microgeometry of gear teeth by adding ease-off, which includes a slope, crown, and end-relief to compensate for distortion of the teeth due to the actual operating conditions. Fortunately, for the gear engineer, there is modern computer software that features 3D graphics that allows the optimization of the ease-off to avoid geometric stress concentration due to edge contact and to obtain minimum Hertzian stress and optimum contact patterns.
Spur Gears
Figure 1 shows a pair of spur gears contacting at the pitch point P at the intersection of the line of centers and the line of action. The red vector is normal to the common tangent line to the contacting tooth profiles. It represents the transmitted force in the zone of single-pair contact. The pitch point P is the only point along the line of action where there is pure rolling contact. At other points of contact, there is a combination of rolling and sliding.
The line of centers is the common connecting line between the two axes of the mating gears, which is the shortest distance between the axes.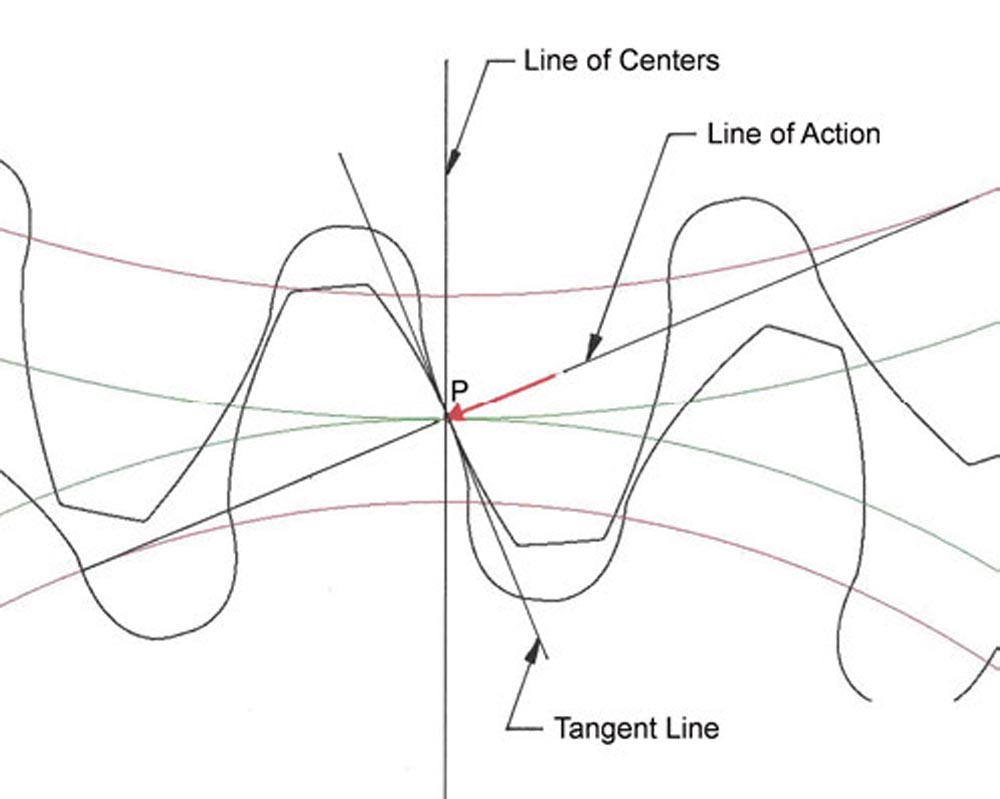
Figure 1—Contact at pitch point P in the transverse plane (Ref. 4).

Figure 2 shows a pair of spur gears contacting at two points in the double-pair zone along the line of action. The red vectors that are normal to the common tangent planes to both contacting tooth profiles represent the transmitted forces in the zone of double-pair contact. With conjugate spur gears these vectors along the common normal at both contact points pass through the pitch point P. The two contact points along the line of action also have tractional forces in the tangent planes due to sliding. The tractional forces are in opposite directions on either side of the line of centers.
If the pitch point remains fixed on the line of centers (the connecting line between the two axes) the velocity ratio (gear ratio) remains constant, and conjugate action is achieved.
In the axial plane, the pitch point P is an axis parallel to the gear axes and is termed the pitch element. The pitch element is the line of tangency between the two pitch cylinders and is the instantaneous axis of relative rotation of the mating gears.
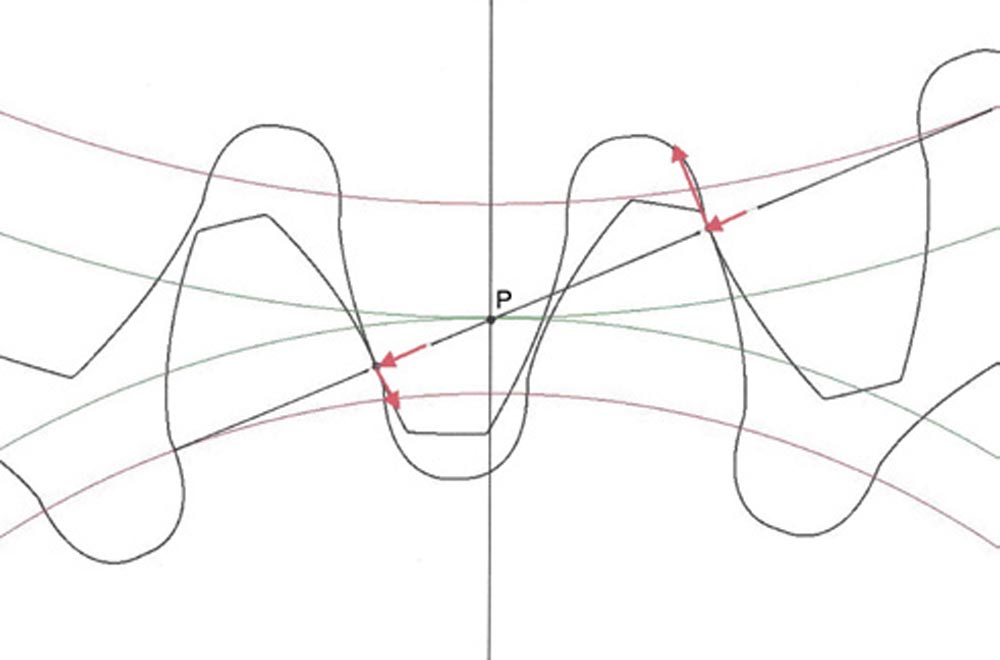

Figure 2—Contact at two points in the double-pair zone in the transverse plane (Ref. 4).
Helical Gears
Figure 3 shows helical gear velocities in the tangent plane. The tangent plane is perpendicular to the contact plane or surface of action. The tangent plane contains the contact line and is perpendicular to the common normal of the contacting teeth. The contact line is located at the intersection of the tangent plane and the contact plane. The red line labeled zo is a general coordinate in the normal plane that locates the contact point Q from pitch point P on the pitch element.
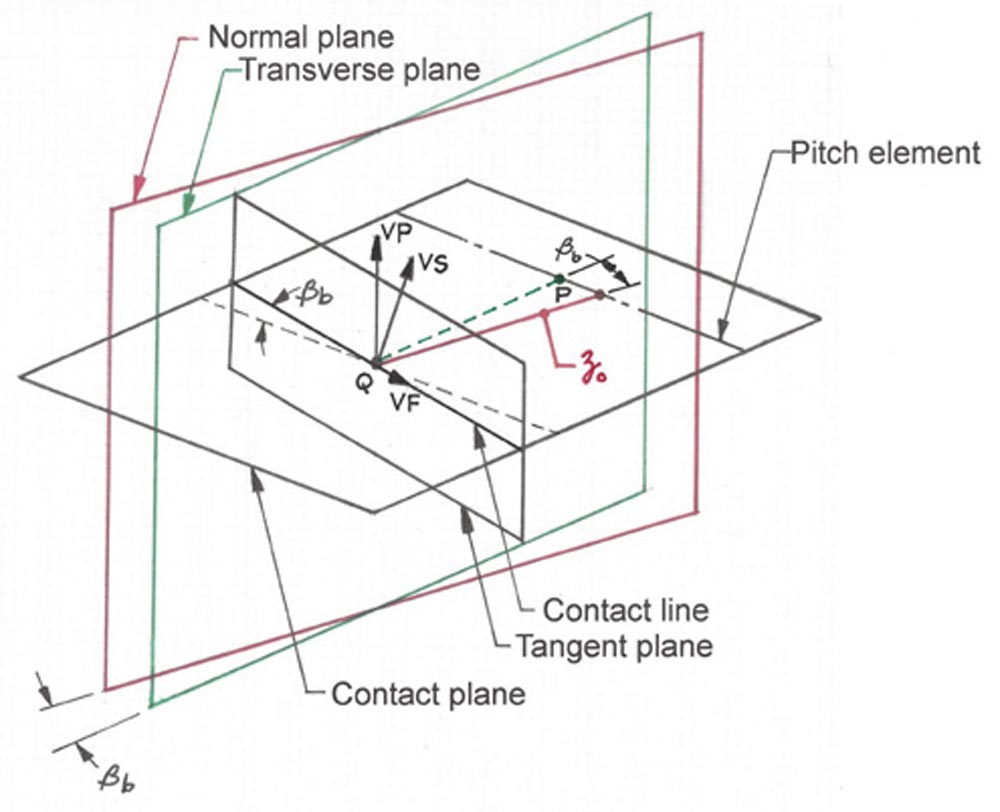
Figure 3—Helical gear velocities in the tangent plane (Ref. 4).
Figure 4 shows contact point Q at a distance zo from pitch point P on the pitch element in the normal plane. Distance zo is a general coordinate, that locates point Q in the normal plane (zo is positive to the left of P and negative to the right of P). Figure 4 shows the contact point Q at the tip of the pinion tooth where the sliding velocities are often maximum.
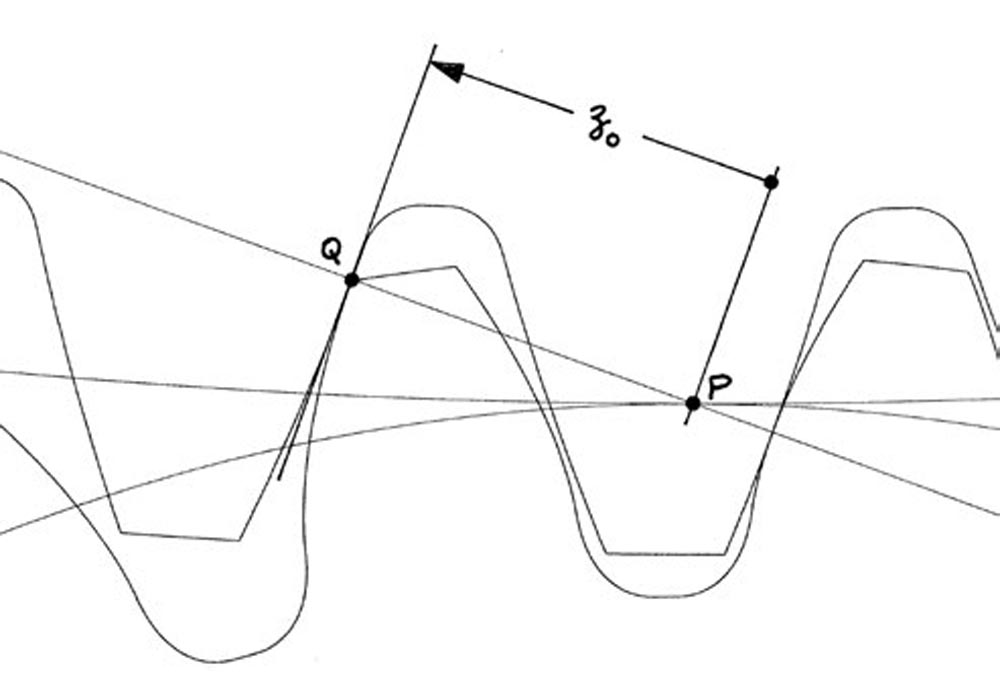
Figure 4—Contact point Q at distance zo from pitch point P in the normal plane (Ref. 3).
Figure 5 shows helical gear sliding velocities VP, VF, and VS at point Q in the tangent plane. It is well known that helical gears have no sliding in the axial direction. However, there is sliding along the contact line in the tangent plane represented by velocity VF.
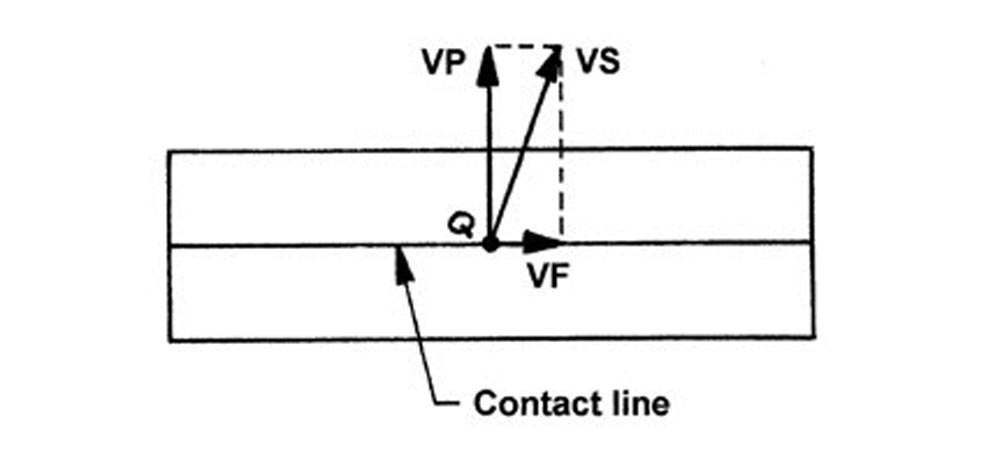
Figure 5—Helical gear velocities VP, VF, and VS at point Q in the tangent plane (Ref. 4).
Table 1 shows sliding velocities calculated with the Mathcad program given in GEARTECH Report (Ref. 4). The equations are from Wells Coleman (Ref. 2). The example is for module 10 helical gears operating at 10,000 rpm and 131 m/s pitch line velocity.
Table 1—Sliding velocities per Refs. 2,4 | |||
β (deg) | VF (m/s) | VP (m/s) | VS (m/s) |
0 | 0.000 | 31.140 | 31.140 |
10 | 1.862 | 30.882 | 30.938 |
15 | 2.800 | 30.551 | 30.679 |
20 | 3.743 | 30.071 | 30.303 |
30 | 5.647 | 28.599 | 29.151 |
45 | 8.471 | 24.767 | 26.176 |
Figure 6 shows how the sliding velocities VF, VP, and VS vary with helix angle. Note that the calculations were performed for point Q at the pinion tooth tip, which is often the point of maximum sliding velocities. However, depending on the pinion profile shift, the point of maximum sliding velocity might be at the other end of the path of contact.
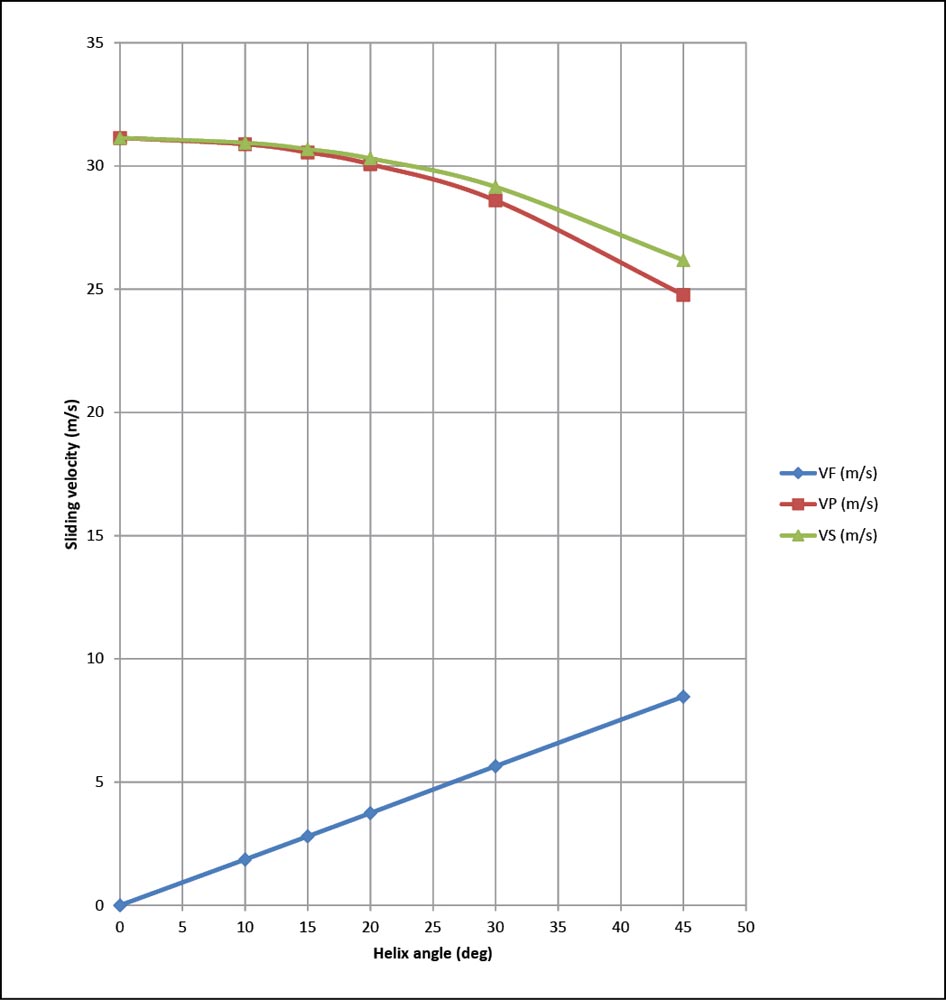
Figure 6—Sliding velocities per reference (Refs. 2,4).
Comparison of Helical Gears and Spur Gears
Helical gearing is essential when tooth-to-tooth transmission smoothness (low transmission error) is required. The smooth running characteristic of helical gears is achieved because all phases of tooth meshing occur simultaneously provided that the face width is large enough to give an axial contact ratio (overlap ratio) greater than unity. In contrast, spur gears have alternating single-pair and double-pair tooth contact that results in much higher transmission error. Furthermore, the resulting stiffness variation causes a nonlinear dynamic response, which increases the risk of resonant vibration that results in much higher dynamic tooth loads. Consequently, audible noise and vibration levels are much higher with spur gears than with helical gears.
Helical gears are capable of very high speeds with pitch line velocity approaching 200 m/s. However, such high speed requires very sophisticated design practice, which includes analyses of temperature rise due to sliding in gears and bearings, windage between gear teeth and the gearbox internal environment, and shearing of lubricant films in gears and bearings. Furthermore, as gear teeth mesh at high speed the air/oil mist in the space between teeth must be expelled by meshing teeth. Helical gears pump the air/oil mist in the axial direction much more efficiently than spur gears. This is the primary reason that spur gears have much lower speed limits than helical gears. On the other hand, the axial pumping velocity of helical gears with low helix angles can be very high (reaching Mach 3), which causes thermal bulging of the gear teeth. Therefore, helical gears with pitch line velocity greater than 100 m/s usually require curvilinear helix modification to compensate for thermal bulging.
Crossed-Axis Helical Gears (Colbourne)
The following analysis is based on Chapter 15, pages 414–429 of Colbourne (Ref. 3).
Requirements for Conjugacy
To be conjugate, the normal base pitches of each crossed-axis helical gear must be the same. This is the only requirement that must be met for conjugacy.
pnb1 = pnb2
Center Distance
The center distance is the line joining the axes, which is perpendicular to both axes and is the shortest distance between the axes. See Figure 7. The center distance is the line C between the points on the axes Co1 and Co2. If an imaginary rack is inserted between the teeth of both gears, it touches the pitch cylinders of the gears in two parallel pitch planes. Crossed-axis helical gears are generally designed so that the values of C and Σ are either equal or approximately equal, to the reference (standard) center distance Cs and reference (standard) shaft angle Σs.
Cs = Rs1 + Rs2
Σs = ψs1 + ψs2
For the particular case where the center distance equals Cs, the shaft angle must equal Σs to maintain conjugacy.
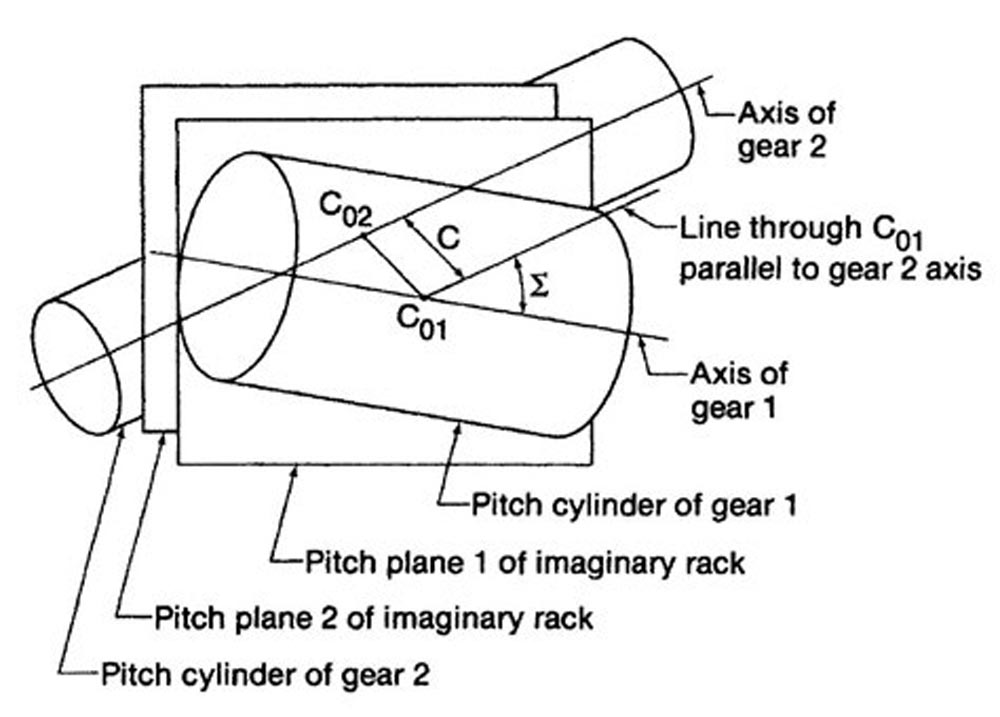
Figure 7—Pitch cylinders in a crossed-helical gear pair (Ref. 3).
Shaft Angle
Figure 8 is a view in the direction of the line of centers. The shaft angle equals the sum of the two working helix angles.
Σ = ψp1 + ψp2
Because crossed-axis helical gears have point contact, they are not sensitive to shaft angle, and they remain conjugate even with small errors in Σ.
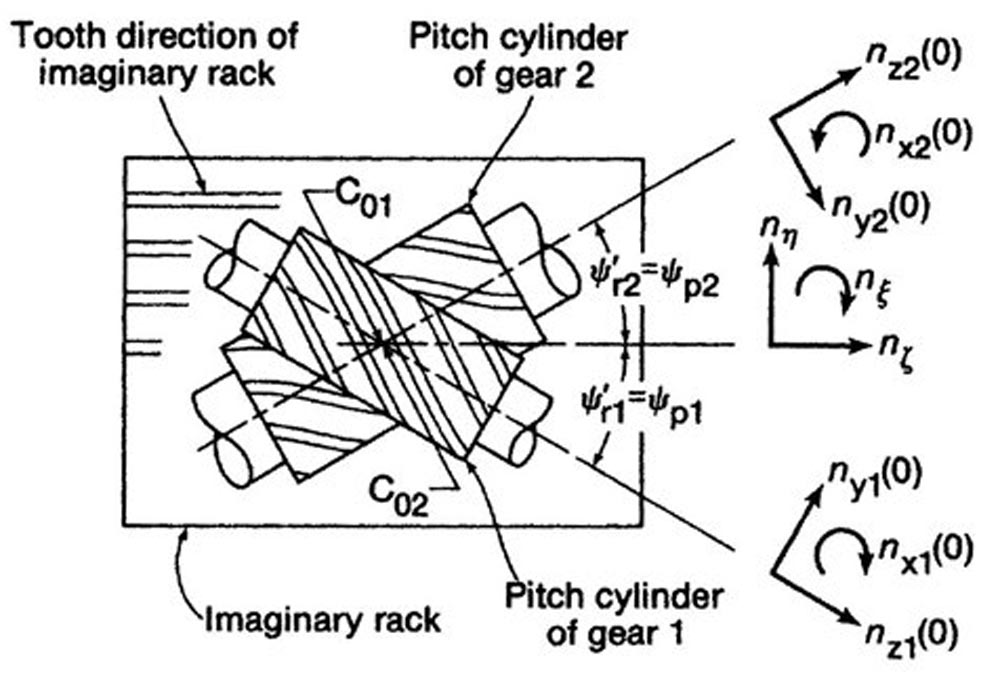
Figure 8—Directions of the unit vectors (Ref. 3).
Pitch Planes
The two pitch cylinders and the imaginary rack pitch planes are shown again in Figure 9, but viewed this time in the negative nη direction, which is perpendicular to the teeth of the imaginary rack.
The center distance is not necessarily equal to the sum of the pitch cylinder radii. Figure 9 shows an example where the pitch planes of the imaginary racks are separated by the distance ΔCp.
ΔCp = C - Rp1 – Rp2
There is no relation between the pitch cylinders and the center distance C, Therefore, the pitch cylinders do not touch, and there is no point of pure rolling.
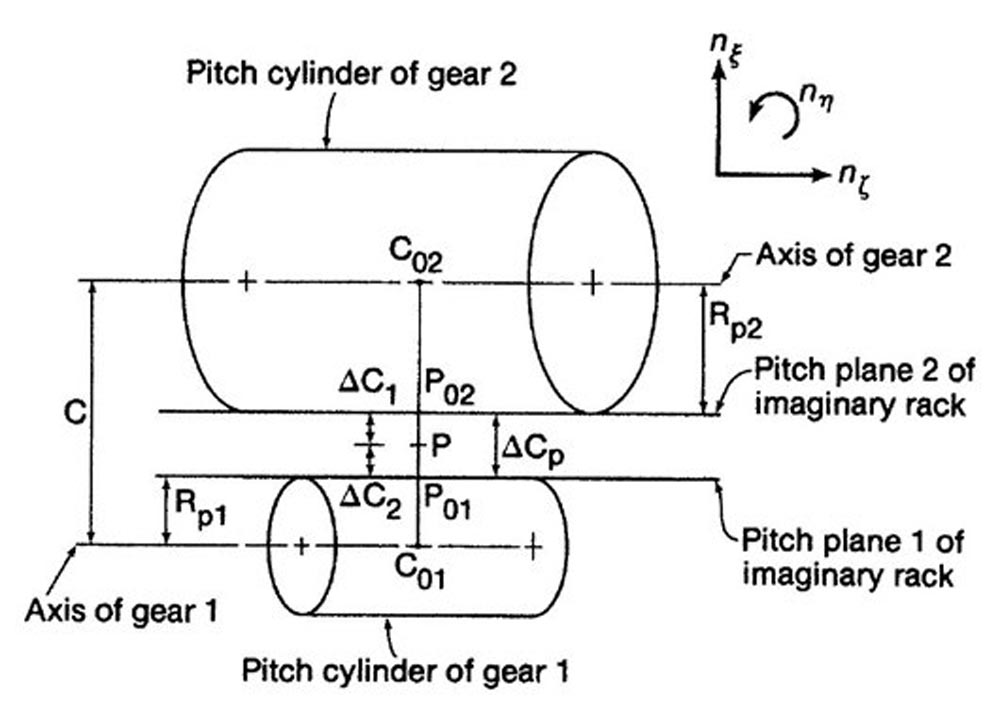
Figure 9—Pitch cylinders perpendicular to teeth of the imaginary rack (Ref. 3).
Pitch Points
Figure 10 shows that the path of contact is a straight line that coincides with the line of action, exactly as it does in the case of a spur gear pair. The path of contact is inclined at the working normal pressure angle to the pitch planes of the imaginary racks and intersects the points P1 and P2 on the pitch planes.
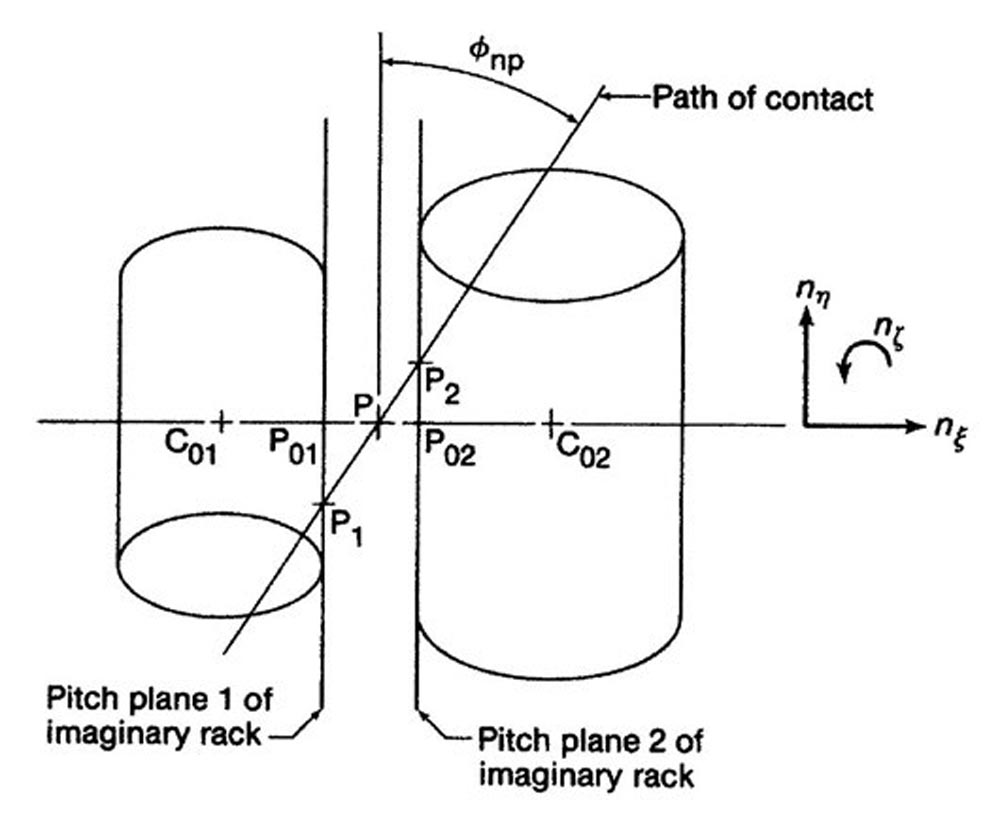
Figure 10—Pitch cylinders viewed along the teeth of the imaginary rack (Ref. 3).
Analogy to a Spur Gear
When the pinion is viewed in the axial direction, as shown in Figure 11, the path of contact appears exactly the same as the path of contact in a spur gear pair. The straight line containing the path of contact extends from a typical contact point A1, passes through the pitch point P1, and touches the base cylinder at point E1.
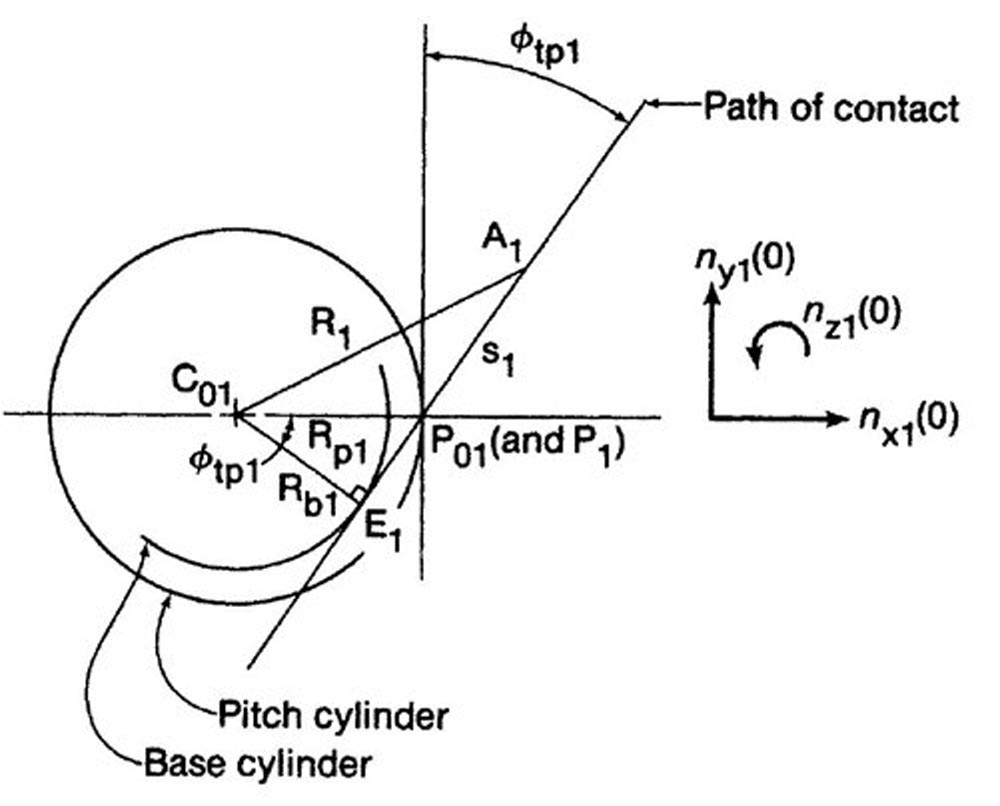
Figure 11—Pitch cylinder of gear 1 viewed along the gear axis (Ref. 3).
Crossed-Axis helical gears (ISO 21771-1:2022)
Figure 12 is based on Figure 32 of ISO 21771-1:2022 (Ref. 5). ISO nomenclature is somewhat different from the nomenclature of this paper. Therefore, certain terms are defined here:
C = P Pitch point in the normal plane
C1 = Pinion pitch point in the transverse plane
C2 = Gear pitch point in the transverse plane
Cn = Pitch point in the tangent plane
Line T1 – T2 = Line of action that passes through the pitch point C = P in the normal plane
Comparison of Helical Gears and Crossed-Axis Helical Gears
Parallel-axis helical gears have contact lines similar to that of parallel cylinders, which take place along straight line generatrices, similar to spur gears, but the lines of contact slope instead of remaining parallel to the axis of the gear. Crossed-axis helical gears have contact lines similar to two cylinders whose axes are not parallel. With axes crossed at 90 degrees, and under near zero load, point contact occurs. At less than a 90-degree shaft angle, and at working load, the contact spreads to an elliptical contact area. Hertzian stress in crossed-axis helical gears with point contact is very much higher than that of parallel-axis helical gears with line contact.
Point contact occurs in crossed-axis helical gears because the straight generatrix of each involute helicoid are tilted relative to each other and cross at a single contact point. With a rotation of the gears, the contact point travels in a straight path of contact in the normal working plane as shown by the line between points A and E in Figure 12.
A high sliding velocity, equal to the vector difference of the two pitch line velocities, is present at all phases of tooth engagement of crossed-axis helical gears. A third vector normal to the other two adds a component of sliding velocity similar to that which occurs in parallel-axis helical gears. This third vector has zero magnitude when contact is at the pitch point and is a maximum when contact is at its furthest point from the pitch point.
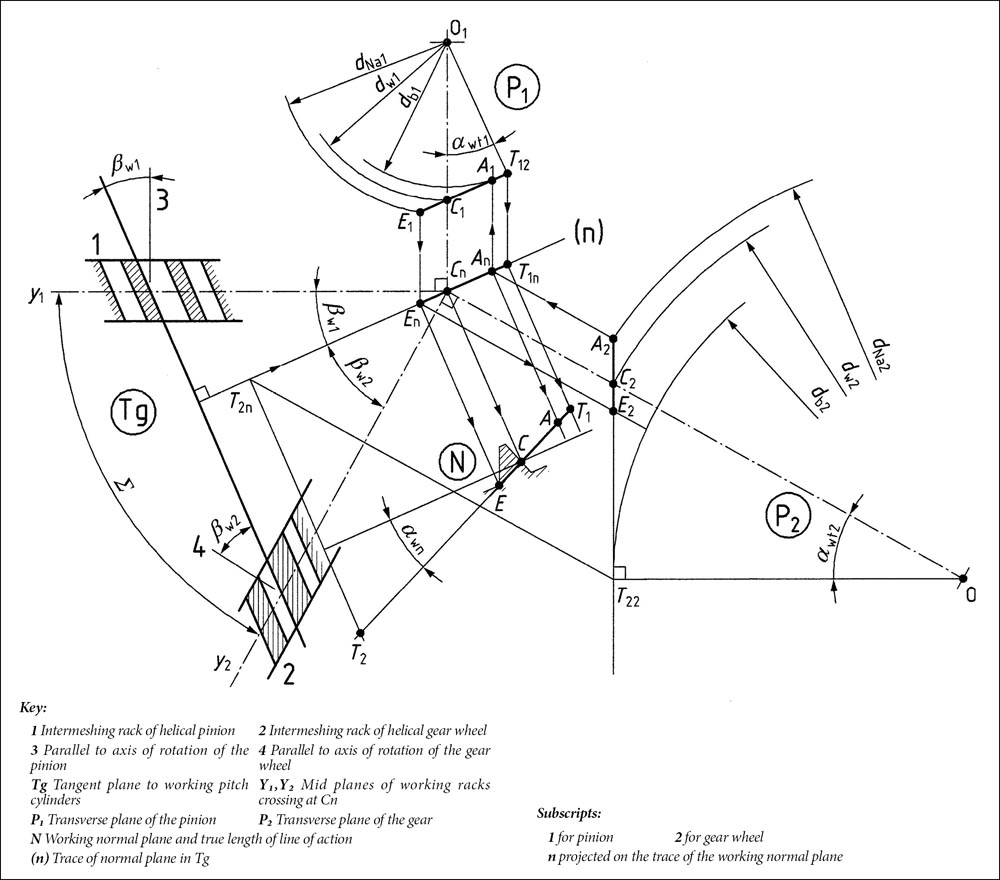
Figure 12—Meshing conditions in transverse planes and working normal plane (Ref. 5).
Because of point contact, crossed-axis helical gears are insensitive to changes in center distance, changes in shaft angle, or changes in the axial position, and they remain conjugate even with reasonably small mounting errors. Furthermore, crossed-axis helical gears are among the easiest to manufacture, which makes them among the least expensive gears. Despite all these advantages, the gear designer must consider that the load capacity of crossed-axis helical gears is severely limited because of the point contact.
Worm Gears
The following three paragraphs are from Buckingham (Ref. 1):
- “The conjugate gear-tooth action between a worm and a worm gear is the same whether the worm is revolved to screw the thread along its axis or whether the worm is moved axially without revolving.”
- “The basic-rack form of the worm gear is the form of that section of the worm thread which actually engages with the worm-gear teeth. This form changes across the face of the worm gear. When these forms are established for any given planes of rotation of the worm gear, conjugate gear tooth forms and trochoidal fillets of the worm gear are determined for these planes of rotation in exactly the same manner as for spur gears.”
- “The essential requirement is that the thread form of the worm and that of the hob or other tool used to generate the worm gear be as nearly identical as possible.”
Buckingham’s statements mean that the conjugacy requirements of worm gearsets are the same as that of a spur gear meshing with its basic rack.
Buckingham (Ref. 6) gives the calculation procedure for contact analysis of worm gear drives in Chapter 11, on pages 240–255. The procedure is as follows:
- The profile of the intersection of the worm thread on planes parallel to the worm axis forms the basic rack profile in the axial plane of the worm.
- Using tangents to the basic rack profiles, coordinates of the path of contact are calculated for each basic rack profile.
- The rack profiles and their paths of contact are then plotted from the calculated data. Where a path of contact intersects its rack profile, it defines one point of contact between the worm and its mating worm gear.
- Several points of contact thus determined are projected into the plan view of the worm to their respective traces of intersecting plane and are then connected with a smooth curve. This curve is the projection of the actual contact line on the plan view of the worm.
- These same points of contact on the rack profiles are also transferred or projected into the end view of the worm to their respective sections. These points, when connected by a smooth curve, give the projection of the actual contact line on the end view of the worm.
The following three figures give the results of an example from Buckingham’s contact analysis of a screw helicoid worm drive (Ref. 6).
Figure 13 shows the end view of a screw helicoid worm. Five axial planes A, B, C, D, and E were selected for the analysis. Distance R1 locates the height of the pitch plane of the worm above its axis. Angles εE and εA are generatrix rotation angles.
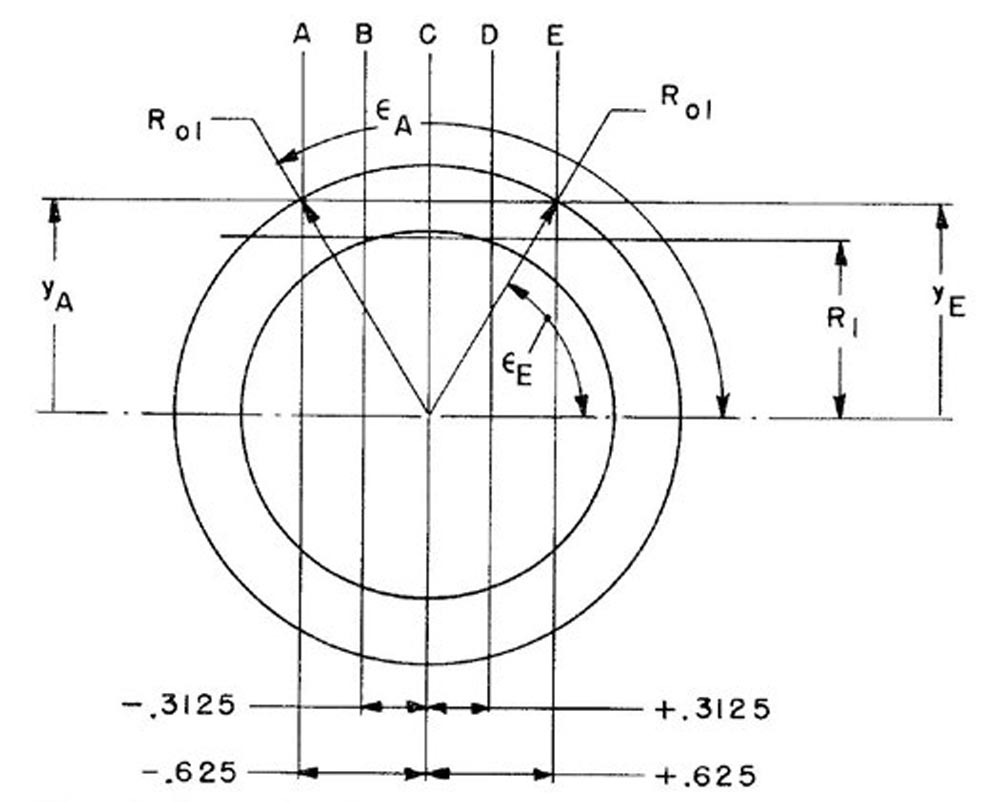
Figure 13—Axial planes, pitch plane, and generatrix rotation angle (Ref. 6).
Figure 14 shows the paths of contact for the axial planes A, C, and E. The rack profile for section C is the generatrix of the helicoid. The intersection between rack profile C and the worm axis is shown after the worm has moved along the worm axis at a distance equal to one-quarter of the lead because it has revolved 90 degrees or one-quarter of the full circle. The intersection of path C with rack profile C locates a contact point between the worm and worm gear. Sections A and E are at the side edges of the worm gear. For section A, the path of contact is outside the rack profile and therefore the contact point is nonexistent. For section E, the contact point is below the pitch plane and beyond the outside radius of the worm gear. Therefore, the contact point is non-existent.
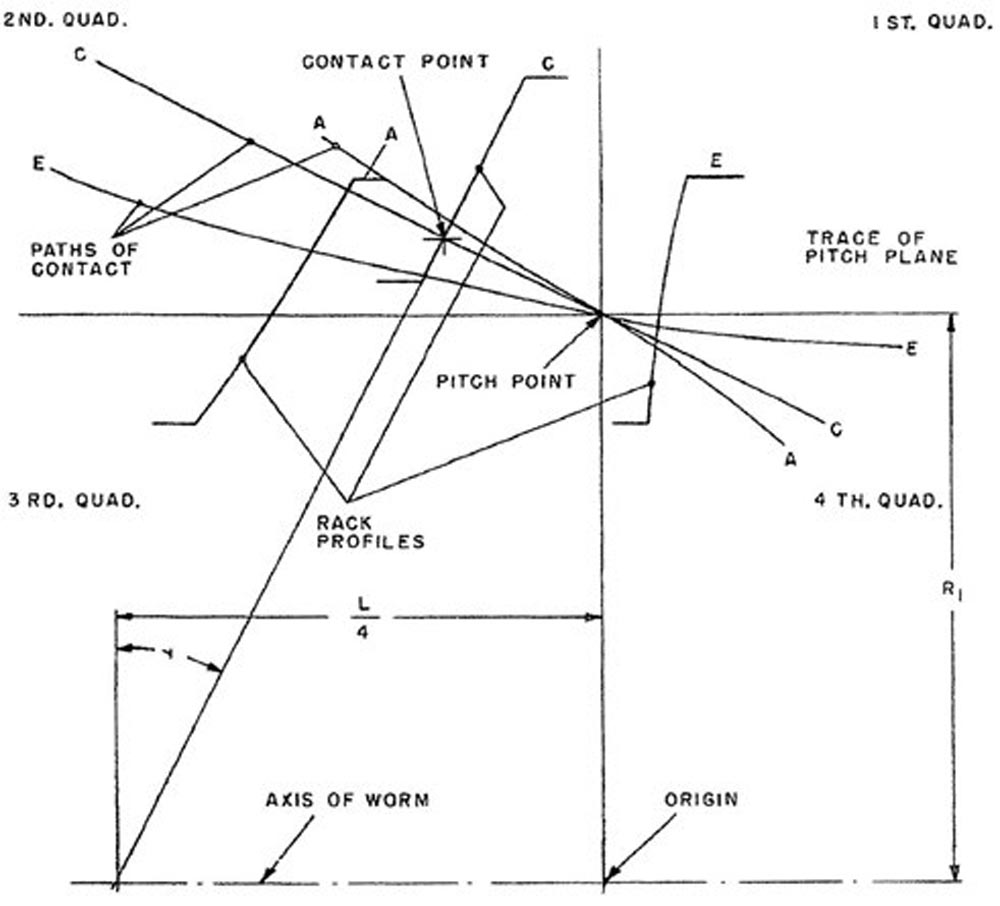
Figure 14—Paths of contact for sections A, C, and E (Ref. 6).
The lower right side of Figure 15 shows a repeat of the Figure 14 paths of contact, but it adds the rack profiles and paths of contact for sections B and D. The outside radius of the worm gear R2, which is also the gear pitch radius, is also shown.
The upper area of Figure 15 shows a plan view and projected field of contact on the worm. The space between the lines marked “root” and “crest” represents the flank of the worm thread on which contact will exist. Contact lines marked 1 and 2 exist on adjacent teeth.
The lower left side of Figure 15 shows the end view of the worm with the projected points from the paths of contact plotted to show the contact lines 1 and 2 on the flanks of adjacent worm teeth.

Figure 15—Paths of contact, the field of contact, and contact lines (Ref. 6).
Comparison of Crossed-Axis Helical Gears and Worm Gears
Crossed-axis helical gears are sometimes confused with worm gears. This is probably because a standard single-start worm can mesh properly with either a standard spur gear or a standard helical gear. When the worm thread is an involute helicoid and the gear is a helical gear, the gear pair is an example of crossed-axis helical gears. There will be true conjugate action, but there will be point contact and low load capacity.
The most common worm gear drive consists of a cylindrical worm (not necessarily with an involute helicoid thread) and an enveloping worm wheel. This is called a single-enveloping worm drive. As discussed in the worm gear section, this combination has line contact in place of the point contact that exists in crossed-axis helical gears. Consequently, a worm drive with a cylindrical worm and an enveloping worm wheel has a much higher load capacity than a crossed-axis helical gear drive. However, many of the advantages of crossed-axis gears such as insensitivity to mounting errors are lost with a worm gear drive.
A double-enveloping worm drive consists of an “hourglass” worm and a throated worm wheel. As the name double-enveloping implies, the worm and the wheel “wrap around” each other. A double-enveloping worm drive has line contact with more teeth in contact and higher load capacity than a single-enveloping worm drive. However, the axial alignment of the worm and wheel in a double-enveloping worm drive is more critical. Furthermore, manufacturing costs are higher than a single-enveloping worm drive.
Bevel Gears (Ref. 7)
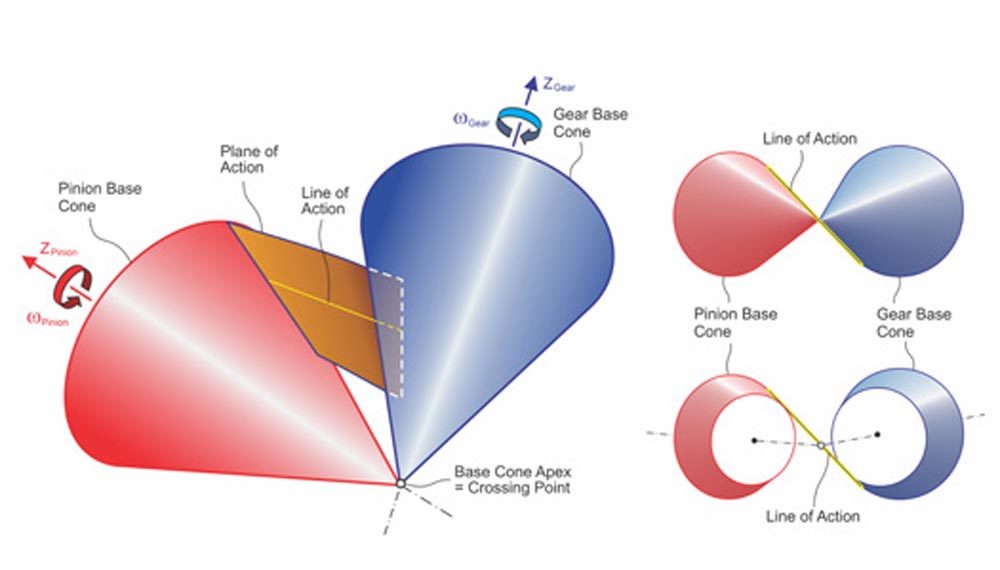
Figure 16 shows the conical pitch surfaces of a bevel gearset. Bevel gears have pitch cones that contact along the pitch element. The pitch apexes coincide at the intersection of the axes of the shafts. The pitch point P is on the connecting line normal to the pitch element and intersects the shaft axes at crossing points no1 – no2.

Figure 16—Bevel shaft axes connecting line, pitch point P, and pitch surfaces (Ref. 7).
Figure 17 shows that the base surfaces of bevel gears are cones whose cone apexes coincide at the intersection of the axes of the shafts. In analogy to cylindrical gears, the line of action is straight and is tangent to the two base circles at a respective location along the width of the cones. If the line of action is shifted along the width of the base cones, the plurality of the lines of action forms the surface of action, which is a plane. This occurs because of the equal and proportional change of the respective base circles while the line of action is shifted. When viewed in the direction of the pitch element, the plane of action appears as a line, like that shown in the two right-side graphics in Figure 17.
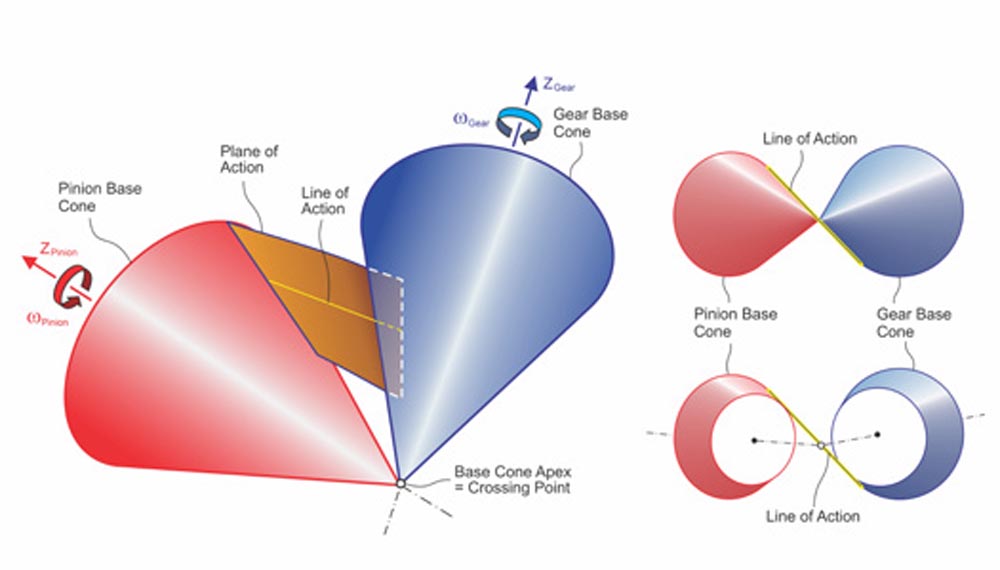
Figure 17—Bevel base cones, the plane of action, and line of action (Ref. 7).
Comparison of Straight Bevel Gears and Spiral Bevel Gears
This comparison is very analogous to the comparison between spur gears and helical gears. The spiral angle results in a face contact ratio larger than zero. The modified contact ratio considers both the transverse contact ratio and the face contact ratio. Typical modified contact ratios of spiral bevel gears are between 2.0 and 2.5. Straight bevel gears cannot achieve modified contact ratios greater than 1.5 because the face contact ratio is always zero. Therefore, spiral bevel gears have a smoother and quieter operation than straight bevel gears.
Straight bevel gears have the significant advantage of the largest possible root thickness for a given module. In the case of bevel gears that are heat treated after cutting but not hard finished, the root bending strength of a spiral bevel gearset would be rather low. The tooth indexing errors resulting from heat treatment distortions reduce the real contact ratio in the operation, so the benefit from the spiral angle diminishes, but the disadvantage from the smaller tooth root thickness remains. This leads manufacturers that do not apply hard finishing to their bevel gears to prefer straight bevel gears.
Straight bevel gears are also preferred in cases when the bearing forces must be low. Many aircraft gear manufacturers apply ground straight bevel gears for the actuation of wing flaps and other components, but they prefer ground spiral bevel gears for power transmission such as the main rotor drives in helicopters.
Hypoid Gears (Ref. 7)
Conjugacy between a hypoid pinion and a hypoid gear is established when the hyperbolic pitch surfaces are designed as explained in Figure 18. The screw axis is the axis of a cylinder, on whose surface the pitch generator line “g” is found as the plurality of points where the shaft connecting line nop – nog passes through the cylinder and is normal to the cylinder. When line “g” is rotated around the pinion axis Za, it generates the pinion pitch surface. When rotated around the gear axis Zb, it generates the gear pitch surface. After one or both pitch surfaces are found, the shaft connecting line nop – nog is added. It describes the location of the pitch point “P” where the connecting line passes through the two contacting pitch surfaces.
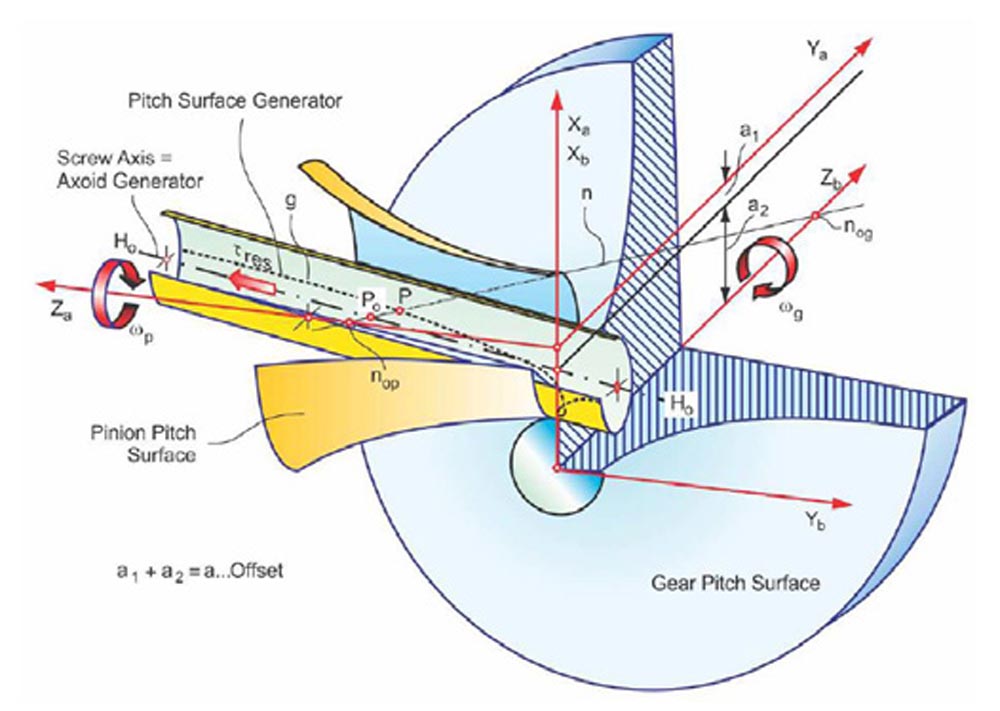
Figure 18—Hypoid shaft connecting line, pitch point P, and pitch surfaces (Ref. 7).
Figure 19 shows the hypoid gear base surface and the surface of action. The surface of action connects the base surface of the pinion and the gear and contacts both base surfaces tangentially. Because the base surfaces are also hyperboloids, the surface of action is not a plane, but a warped surface.
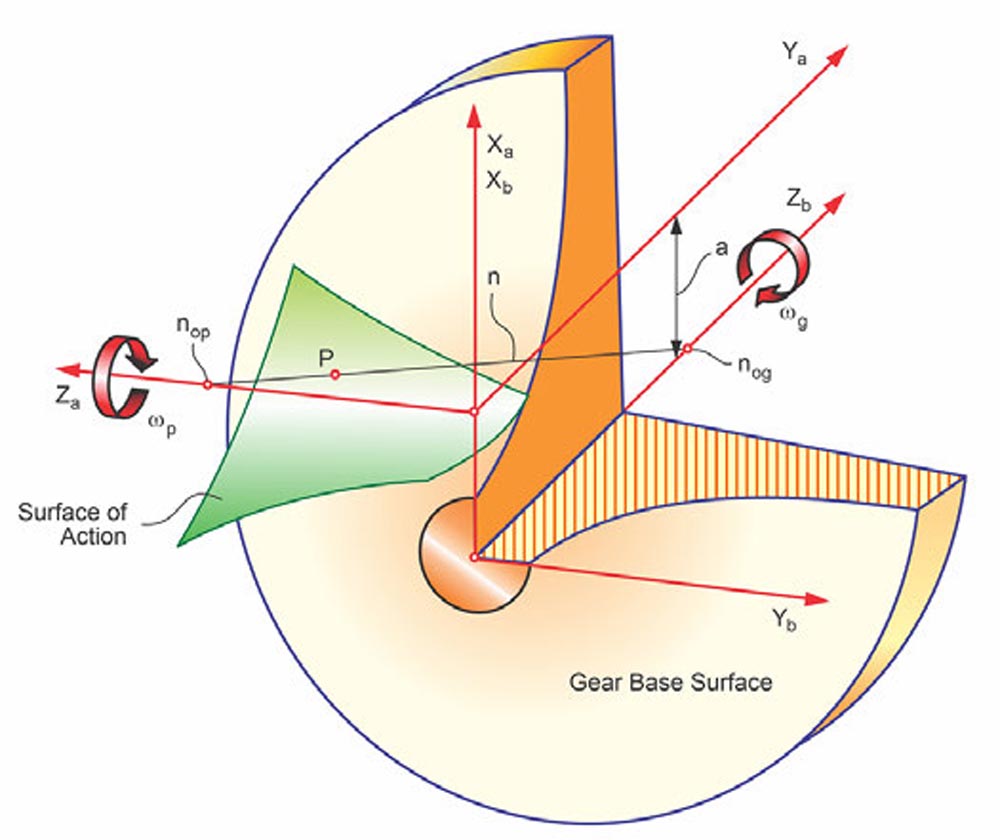
Figure 19—Hypoid gear pitch surface and surface of action (Ref. 7).
Comparison of Bevel Gears and Hypoid Gears
The original intention of the development of hypoid gears by Ernest Wildhaber was to provide the additional design freedom of a pinion shaft offset in bevel gearing. This freedom allowed the automotive manufacturer in the 1920s to lower the bodies of their vehicles by up to two inches. The lower center of gravity of these vehicles provided more safety and better handling in the early vehicles. Still, today, rear-wheel and all-wheel drive vehicles use hypoid gears for this reason.
The hypoid offset increases the pinion spiral angle, which leads to a pinion diameter increase of up to twice the diameter of a spiral bevel pinion. The size increase strengthens the hypoid pinion, which for spiral bevel gears is the weaker of the two members. In addition to the sliding velocities in the profile direction, hypoid gears have a screw motion between pinion and gear, which creates sliding velocities in the face-width direction. These lengthwise sliding motions can be two to three times in magnitude compared to the profile sliding. The lengthwise sliding provides several advantages. The elastohydrodynamic lubrication film is not compromised at the pitch line, where spiral bevel gears exhibit pure rolling and no sliding. The lengthwise sliding also provides a dampening effect, which in combination with the larger pinion spiral angle makes hypoid gearsets operate quieter.
The disadvantage of lengthwise sliding is that it limits the pitch line velocity of hypoids. While spiral bevel gears are used in aircraft applications well above 120 m/s pitch line velocity, hypoid gears, depending on the hypoid offset, are limited to about 60 m/s. A further disadvantage for hypoid gears is that the lengthwise sliding creates the risk of rippling, ridging, or scuffing failure modes. This is the reason that hypoid rear axle drives require lubricants with antiscuff additives.
The manufacturing process of spiral bevel and hypoid gears is identical. The same machines and cutting tools are used for both bevel gear types. In cases where the hard finishing process is lapping, hypoid gears have an advantage. The additional lengthwise sliding of hypoid gears provides a more effective and uniform material removal during lapping. Spiral bevel gears show no material removal by lapping along the pitch line, but lapping grit is often pressed into the surface at the pitch line and remains there, which can cause surface damage such as pitting in a medium to high load operation.
Significance of Conjugacy and Conclusions
Conjugacy is a good basis for gear design, but conjugate gears are not practical for typical gear applications where operational loads, speeds, temperatures, and manufacturing deviations cause displacements of the gear teeth that disrupt conjugate action. The displacements include:
- Elastic deflections of the pinion and gear teeth, shafts, bearings, housings, and foundations due to applied loads.
- Centrifugal distortion of pinion and gear teeth and gear bodies due to high speeds.
- Thermal expansion and distortions caused by power losses due to sliding in gears and bearings, windage between gear teeth and the gearbox internal environment, and shearing of lubricant films in gears and bearings.
- Rigid body displacements of the pinion and gear teeth, shafts, bearings, housings, and foundations due to manufacturing deviations and assembly clearances.
Consequently, modern gear teeth are designed to compensate for all the above displacements that disrupt conjugate action by modifying the profile and helix of gear teeth to have a combination of slope, crown, and end relief such that the gears are as conjugate as possible under the actual operating conditions.

References
- Earle Buckingham, Analytical Mechanics of Gears, McGraw-Hill, 1949.
- Wells Coleman, “Interdisciplinary Approach to the Lubrication of Concentrated Contacts: Gear Design Considerations,” Presented at NASA Symposium Rensselaer Polytechnic Institute, Troy, NY, July 15–17, 1969.
- John Colbourne, The Geometry of Involute Gears, Springer-Verlag, 1987.
- GEARTECH Report NOM-11, “Spur and Helical Gear Velocities,” April 2, 2021, Rev A.
- ISO 21771-1:2022, “Gears-Cylindrical Involute Gears and Gear Pairs- Concepts and Geometry,” Draft of 2022-07-20 (under development).
- Earle Buckingham and Henry H. Ryffel, Design of Worm and Spiral Gears: A Manual for the Design and Manufacture of All-recess-action Worm and Spiral Gear Drives, Buckingham Associates, Inc., 1973.
- Hermann Stadtfeld, “Why are Today’s Hypoids the Perfect Crossed-Axes Gear Pairs?” Gear Solutions, May, 2019.